Relationship between Rate Distance And Time Example
Rate, distance, and time are interrelated in a simple equation: rate = distance ÷ time. For example, if you travel 60 miles in 2 hours, your rate of travel is 30 miles per hour.
Understanding this relationship is crucial for various activities such as driving, running, or cycling, as it helps in calculating the speed at which one is moving. By knowing the relationship between rate, distance, and time, individuals can make informed decisions about travel and transportation.
This knowledge also plays a vital role in fields like physics, engineering, and sports, where the concept of speed and distance is crucial. Understanding this relationship can help individuals become more conscious and efficient in managing their time and travel activities.
Understanding Rate, Distance, And Time
Understanding Rate, Distance, and Time is imperative for grasping the fundamentals of physics and real-life scenarios. The relationship among these three factors plays a vital role in various fields, from transportation logistics to athletic training. By understanding the dynamics between rate, distance, and time, one can effectively calculate speed, plan journeys, and comprehend the impact of varying velocities.
Defining The Key Concepts
Before delving into the intricate connection between rate, distance, and time, it’s essential to define each concept.
- Rate: The rate relates to the speed at which an object moves or an event occurs, typically denoted in units such as miles per hour or meters per second.
- Distance: Distance refers to the measurement of space between two points, indicated in units like kilometers, miles, or meters.
- Time: Time represents the duration or interval during which an action takes place, measured in hours, minutes, seconds, etc.
Exploring The Relationship Between The Three Factors
When any two of these factors are known, the third can be easily determined through simple manipulations of the formula. For example, if one knows the distance traveled and the time it took, the speed can be calculated. Similarly, if the speed and time are given, one can find the distance covered.
Rate, Distance, And Time Equation
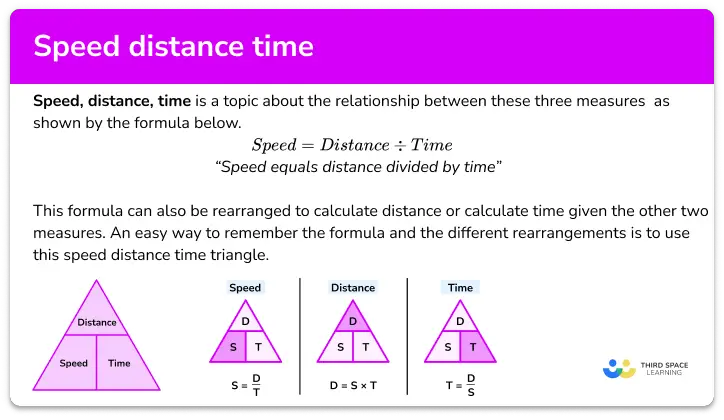
The Rate, Distance, and Time equation is a fundamental concept in physics and mathematics that describes the relationship between the speed at which an object is moving, the distance it travels, and the time it takes to travel that distance. Understanding this equation is essential for solving various practical problems that involve motion, such as calculating the time it takes for a journey, determining the speed of a moving object, or estimating the distance covered.
Formulating The Mathematical Relationship
When dealing with the relationship between rate, distance, and time, the following equation serves as the foundation:
- D = Distance traveled
- R = Rate or speed of the object
- T = Time taken to travel the distance
This simple equation allows us to express the connection between these three factors, providing a framework for solving problems related to motion and travel.
Example Of The Equation In Practical Scenarios
| Rate (R) | Time (T) | Distance (D) |
|---|---|---|
| 60 miles per hour | 2.5 hours | 150 miles |
In this scenario, the car will cover a distance of 150 miles in 2.5 hours at a constant speed of 60 miles per hour. This calculation demonstrates the practical use of the rate, distance, and time equation in real-world situations.
Real-life Examples
Explore real-life examples of the relationship between rate, distance, and time. Discover how these elements are interconnected to determine various scenarios in everyday situations, illuminating the practical application of these concepts in understanding the dynamics of travel and movement.
Real-life Examples The relationship between rate, distance, and time can be easily understood through real-life examples. By seeing how these elements interact in everyday situations and case studies, we can gain a better grasp of their interconnectedness. Illustrating the relationship in everyday situations In our daily lives, the relationship between rate, distance, and time is constantly at play. Consider the simple act of driving a car. The faster you drive, the more distance you cover in a given amount of time. If you are traveling at a speed of 60 miles per hour, you will cover a distance of 60 miles in just 1 hour. Conversely, if you decrease your speed to 30 miles per hour, it will take twice as long to cover the same 60-mile distance. This demonstrates the direct correlation between speed, distance, and time. Case studies showcasing the interplay of rate, distance, and time Let’s delve into some case studies that showcase the interplay of rate, distance, and time. 1. Case Study 1: The Express vs. Local Train Imagine you have two trains traveling from point A to point B. The express train runs at a speed of 100 miles per hour, while the local train travels at 60 miles per hour. The distance between the two points is 300 miles. The express train will cover the distance in 3 hours, while the local train will take 5 hours to complete the same journey. This demonstrates how rate directly affects the time taken to cover a specific distance. 2. Case Study 2: Delivery Services Consider two delivery services transporting goods from one city to another. Service A covers a distance of 200 miles at an average speed of 50 miles per hour, whereas Service B travels the same distance at an average speed of 75 miles per hour. Service A takes 4 hours to complete the delivery, while Service B completes the task in 2.67 hours. This real-world example highlights how varying rates influence the time required to cover a specific distance. By examining these examples, we can see how the relationship between rate, distance, and time is not only relevant in real-life scenarios but also holds crucial implications for various activities and industries.Importance In Various Fields
The relationship between rate, distance, and time holds immense importance in various fields, offering valuable insights and applications across different industries. Analyzing this relationship can provide significant advantages in physics, engineering, and economics, ultimately impacting the efficiency and effectiveness of diverse sectors.
Analyzing The Significance In Physics
In the realm of physics, the understanding of the relationship between rate, distance, and time is fundamental for a wide range of concepts and calculations. This understanding facilitates the analysis of motion, velocity, and acceleration, forming the core of many physical principles and laws. It enables physicists to accurately predict and describe the movements of objects and systems, serving as a crucial foundation for numerous experiments and research endeavors.
Importance In Engineering
For engineers, the relationship between rate, distance, and time plays a pivotal role in the design and optimization of various systems and processes. It is indispensable in the development of transportation technologies, such as cars, airplanes, and trains, where precise calculations of speed, distance traveled, and travel time are essential. Moreover, in the context of manufacturing and production, this relationship guides efficiency improvements and resource management, influencing the overall productivity of industrial operations.
Significance In Economics
In the field of economics, the understanding of rate, distance, and time is crucial for analyzing market dynamics, supply chain logistics, and consumer behavior. It forms the basis for cost-benefit analyses, pricing strategies, and investment decisions. By comprehending this relationship, economists can assess the impact of transportation costs, delivery times, and production rates on the profitability and sustainability of businesses, contributing to informed and strategic economic planning.
Tips For Solving Problems
When it comes to understanding the relationship between rate, distance, and time, having the right strategies can make problem-solving easier. By effectively utilizing the concept of rate, distance, and time and being aware of common pitfalls, you can tackle problems with confidence. Let’s explore some valuable tips for solving problems related to rate, distance, and time.
Strategies For Effectively Utilizing The Rate, Distance, And Time Concept
One of the key strategies for effectively utilizing the rate, distance, and time concept is to understand the interdependency of these three variables. Creating a solid foundation in comprehending how changes in one variable affect the others will significantly enhance problem-solving skills. Below are some key strategies:
- Know the formulas: Familiarize yourself with the fundamental formulas such as Distance = Rate × Time and Rate = Distance / Time. Understanding these formulas will help you manipulate the variables effectively.
- Work with consistent units: Ensure that all the units for rate, distance, and time are consistent. This helps in avoiding errors and simplifies calculations.
- Use visual aids: Visualization can be a powerful tool in understanding the relationships between rate, distance, and time. Graphs, tables, and diagrams can help in making complex problems more manageable.
Common Pitfalls And How To Avoid Them
Understanding the common pitfalls associated with rate, distance, and time problems is crucial for overcoming challenges. By recognizing these pitfalls, you can take proactive measures to avoid them. Here are some common pitfalls and ways to steer clear of them:
- Incorrect unit conversion: One common pitfall is using inconsistent units when dealing with rate, distance, and time. Always double-check and ensure that the units are converted to the same measurement system before proceeding with calculations.
- Overlooking relative motion: Problems involving multiple objects moving relative to each other can be tricky. Avoid the pitfall of overlooking relative motion by carefully analyzing the direction and speed of each object.
- Assuming constant rate or time: Not all scenarios involve constant rate or time. Be cautious of assuming constant values in situations where rate and time may vary.
Frequently Asked Questions For Relationship Between Rate Distance And Time Example
What Is The Relationship Between Rate, Distance, And Time?
When a vehicle travels at a constant rate, the distance covered is directly proportional to the time taken.
How Can Rate, Distance, And Time Be Calculated?
You can calculate rate by dividing distance by time and distance by multiplying rate by time.
Why Is The Relationship Between Rate, Distance, And Time Important?
Understanding this relationship helps in planning travel time and expenses more accurately.
What Are The Units Used To Measure Rate, Distance, And Time?
Rate is measured in units like miles per hour, distance in miles, and time in hours or minutes.
Can Rate, Distance, And Time Be Used In Real-life Scenarios?
Yes, these concepts are used in everyday situations such as commuting, sports, and logistics.
How Can One Improve Their Understanding Of Rate, Distance, And Time?
Practicing word problems and visualizing scenarios can enhance your understanding of these concepts.
Conclusion
Understanding the relationship between rate, distance, and time is crucial for various real-life applications. By grasping the simple formulas and concepts presented in this blog post, you can efficiently solve problems related to speed, travel distance, and time taken. This fundamental knowledge will undoubtedly enhance your analytical and problem-solving skills in everyday situations.